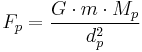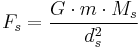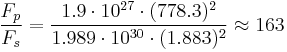Tug of war (astronomy)

The tug of war in astronomy is the ratio of planetary and solar attractions on a natural satellite. The term was coined by Isaac Asimov in The Magazine of Fantasy and Science Fiction in 1963. ^[1]

1 Law of universal gravitation
2 Example
3 The table of planets
4 The special case of Moon
5 References

Law of universal gravitation

According to Isaac Newton's law of universal gravitation

$F= G\cdot \frac{m_1 \cdot m_2} {d^2}$

In this equation

F is the force of attraction

G is the gravitational constant

m₁ and m₂ are the masses of two bodies

d is the distance of separation between the two bodies

The two main attraction forces on a satellite are the attraction of the sun and the primary (the planet around which the satellite revolves) Thus the two forces are

$F_p= \frac{G \cdot m \cdot M_p} {d_p^2}$

$F_s= \frac{G \cdot m \cdot M_s} {d_s^2}$

where the subscripts p and s represent the primary and the sun respectively.

The ratio of the two is

$\frac{F_p}{F_s} = \frac{M_p \cdot d_s^2}{M_s \cdot d_p^2}$

Example

Callisto is a satellite of Jupiter. The parameters in the equation are ^[2]

Callisto-Jupiter distance (d_p) is 1.883 · 10⁶ km.
Mass of Jupiter (M_p) is 1.9 · 10²⁷ kg
Jupiter-Sun distance (ie, mean distance of Callisto from the Sun, m_s) is 778.3 · 10⁶ km.
The solar mass (M_s) is 1.989 · 10³⁰ kg

$\frac{F_p}{F_s} = \frac{1.9 \cdot 10^{27} \cdot (778.3)^2}{1.989 \cdot 10^{30} \cdot(1.883)^2} \approx 163$

The table of planets

Asimov lists tug of war ratio for 32 satellites (then known in 1963) of Solar system. The list below shows one example from each planet.

Primary	Satellite	Tug of War ratio
Neptune	Triton	8400
Uranus	Titania	1750
Saturn	Titan	380
Jupiter	Ganymede	490
Mars	Phobos	195
Earth	Moon	0.46

The special case of Moon

Unlike other satellites of the solar system solar attraction on Moon is more than that of its primary. According to Asimov, Moon is a planet moving around the Sun in careful step with the Earth.^[1]

References

^ ^a ^b Isaac Asimov: Asimov on Astronomy Coronet Books,1976, ISBN 0-340-20015-4 pp125-139
^ Thomas Arny: Explorations, Mc Graw Hill, ISBN 0-07-561112-0 pp.543-545